We have developed a first-order analytical solution for describing circular orbital motion. Projecting the perturbations onto a topocentric reference frame yields a literal analytical expression for the radial velocity (Doppler signal) as a function of the geopotential coefficients. We also mapped the sensitivity of the signal to inadequate knowledge of Earth's gravity field, as characterised by the differences between the JGM-3 and GRIM4-S4 models. On average the signal is 0.2 mm/s for the TOPEX /POSEIDON orbit and 0.7 mm/s for Spot.

Analysing satellite orbital perturbations is important to the preparation and operation of earth observation missions. Analysing the errors, especially on the radial and tangential components of satellite motion, is an extension to this type of work. This is particularly true in satellite altimetry, where orbit error determination can become an area of study in its own right. Analysis can be based on observation data, or can be done theoretically, going back to the errors assigned to geopotential coefficients. It can even be done using both types of approach (e.g. Schrama, 1992). We chose to analyse orbital error theoretically. This meant using an analyt-ical theory of the satellite motion and an estimate of the uncertainty on our knowledge of Earth's gravity field. The aim was to produce a tool able to realistically derive the sensitivity of the satellite position, and even the variation of its position relative to an ob-server on Earth, to the error on the gravity field coefficients.

The representation of orbital perturbations is essentially based on analytical theories using Kepler elements (Kaula, 1966). However, satellite missions often require perturbations in coordinate form, as is the case with altimetry. Recently, interest in this type of work has increased significantly because of the need for an accurate error budget, at least on the radial component, of the TOPEX/ POSEIDON (T/P) orbit.

In space geodesy applications, the benefits of using coordinates, typically non-singular variables, in developing an analytical satellite motion theory are twofold. First, avoiding singular variables, as many earth observation missions are on circular or near-circular orbits. Second, it is possible to obtain literal analytical expressions, in coordinate form, for simpler, more effective analysis of the perturbations on the satellite position. For example, Hill's equations have been used to obtain the radial and normal orbital perturbations due to the geopotential (Schrama, 1989; Balmino et al., 1996). However, we recently showed the benefits of using spherical geocentric coordinates for developing a new analytical solution to the problem of perturbed circular motion. These are clearly more suitable than local coordinates for describing perturbations of grav-itational origin (Exertier and Bonnefond, 1996).

Using literal analytical expressions for the perturbations in coordinates, as supplied by analytical theory, the orbital error still needs estimating correctly. One of the inputs is the gravity field model variance/covariance matrix (Balmino, 1992). However, it is still difficult to handle numerically, and the propagation of the associated radial orbit error is only estimated.
We therefore decided to investigate the error on the gravity field coefficients based on the differences between recent models: GRIM4, JGM2 and JGM3. This has the advantages of simple formulation and being usable within linear perturbation theory. On the other hand, as the error assessment does not take account of the correlations between the harmonic coefficients, it is clearly pessimistic. One way of checking it is to compare the results of the theoret-ical prediction of orbit error with recent assessments based on data.

An immediate application of analytical theory consisted of describing the differences in TOPEX/POSEIDON radial orbits (dr) linked to the differences (dC_l.m, dS_l.m) between the JGM-3 and GRIM4 models. These pseudo-errors, projected along the ground tracks of a ten-day ephemeris, yield the "geographically-correlated" errors. Theory predicts deviations of up to 2.5 cm rms for the radial component. As a check, an equivalent geograph-ical distribution and equivalent amplitudes have been found numerically from GPS data (Haines et al., 1995). This confirms the usefulness and effectiveness of the analytical method, which has direct applications.

We would now like to discuss an extension of this early work.
Using analytical expressions of the perturbations expressed in geocentric coordinates, the perturbations can be projected onto any given topocentric reference frame. The aim is to derive a direct analytical relationship, still in the form of first-order approximations, between the variation in the satellite position (radial velocity) relative to a station and the perturbations, themselves linked to the gravity field coefficients. This makes it possible to directly describe the error on the radial velocity, as linked to the Doppler signal, from the current uncertainties (dC_l.m, dS_l.m) on our knowledge of Earth's gravity field. The satellite orbit becomes simply an intermediate stage in the calculation, expressing the integration of phenom-ena at the desired altitude. The effects are then described in a very different form from conventional orbit error.

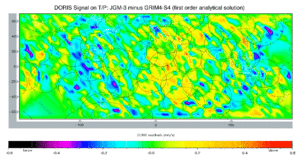
Figure 1 shows, for the DORIS station network and TOPEX/POSEIDON orbit, the error predictions in terms of radial velocity - for the DORIS Doppler signal, for example - due to a differ-ence between the coefficients of the JGM-3 and GRIM4-S4 models.
The signal is calculated by stations along the satellite tracks over a ten-day period, i.e. for all passes above 25 elevation. The visibility circles show where the signal is actually calculated. At large scales, the features are well correlated with corresponding uncertainties on Earth's gravity field. Their amplitude is 0.2 mm/s rms, with deviations at 0.8 mm/s. A second application (Figure 2), for the SPOT orbit, shows a stronger signal, 0.7 mm/s with deviations at 3.5 mm/s.

Conclusion

We have developed an analytical solution for perturbed circular motion, as a first-order approximation.
In space geodesy projects, the main application of such a solution, expressed in coordinates, is for analysing orbital perturbations and the associated errors.

Using literal expressions for the perturbations and the differences in coefficients between recent gravity field models, we predict TOPEX/POSEIDON orbit radial error to be around 2.5 cm rms. The analytical solution can be extended to project perturbation expressions onto a topocentric reference frame.
This makes it possible to describe the signature of differences between gravity field models in terms of radial velocity "residuals".

Through the results we attempt to show that a Doppler signal such as from DORIS in its present form, with measurement errors currently around 0.3 mm/s, can provide no more extra information, on average, on Earth's gravity field (GRIM4 model, for example) from the TOPEX/POSEIDON orbital altitude. To improve the gravity field using tracking measurements, we need to progress toward 0.1 mm/s instrumentation in similar future missions, e.g. Jason. For TOPEX/POSEIDON, inadequate knowledge of the gravity field is no longer the dominant source of orbital error. We need to look elsewhere for the cause of the current 0.55 mm/s rms error in orbital calculations, e.g. propagation errors, non-gravitational errors, station siting, etc. The situation is obviously quite differ-ent for SPOT-type orbits, at 800 km altitude.

References

• Balmino, G. (1992): Orbit Choice and the Theory of Radial Orbit Error for Altimetry, International Summer School of Theoretical Geodesy, ''Satellite Altimetry in Geodesy and Oceanography'', Lecture Notes, Trieste, Italy, May 25-June 6
• Balmino, G., E. Schrama and N. Sneeuw (1996): Compatibility of first-order circular orbit perturbations theories; consequences for cross-track inclination functions, Journal of Geodesy, 70:554--561.
• Exertier, P. and P. Bonnefond (1996): Analytical Solution of Perturbed Circular Motion: Application to Satellite Geodesy, à paraître dans Journal of Geodesy.
• Haines, B.J., E.J. Christensen, J.R. Guinn, R.A. Norman and A. Marshall (1995): Observations of TOPEX/POSEIDON Orbit Errors Due to Gravitationnal and Tidal Modeling Errors Using the Global Positioning System, IUGG General Assembly, Jul 3-15, Boulder, CO
• Kaula, W. (1966): Theory of Satellite Geodesy, Blaisdell, Waltham, Mass
• Schrama, E.J.O. (1989): The Role of Orbit Errors in Processing of Satellite Altimeter Data, Netherlands Geodetic Commission, report 33
• Schrama, E.J.O. (1992): Some remarks on several definitions of geographically correlated orbit errors: consequences for satellite altimetry, Manuscripta Geodaetica, 17:282--294